백준 1493번 (박스 채우기, C++, 분할 정복) [BAEKJOON]

글쓴이 / / BaekjoonOnlineJudge, C++/CPP
목차 테이블
  1. 박스 채우기
  2. 입력 확인
  3. 잘못된 로직과 코드 1
  4. 잘못된 로직과 코드 2
  5. 잘못된 로직과 코드 3
  6. 통과된 코드

박스 채우기

www.acmicpc.net/problem/1493

시간 제한메모리 제한제출정답맞힌 사람정답 비율
2 초128 MB4718127190927.224%

문제

세준이는 length × width × height 크기의 박스를 가지고 있다.

그리고 세준이는 이 박스를 큐브를 이용해서 채우려고 한다.

큐브는 정육면체 모양이며, 한 변의 길이는 2의 제곱꼴이다.

(1×1×1, 2×2×2, 4×4×4, 8×8×8, …)

세준이가 가지고 있는 박스의 종류와 큐브의 종류와 개수가 주어졌을 때,

박스를 채우는데 필요한 큐브의 최소 개수를 출력하는 프로그램을 작성하시오.

입력

첫째 줄에 세 자연수 length width height가 주어진다.

둘째 줄에 세준이가 가지고 있는 큐브의 종류의 개수 N이 주어진다.

셋째 줄부터 총 N개의 줄에 큐브의 종류 Ai와 개수 Bi가 i가 증가하는 순서대로 주어진다.

큐브의 종류는 한 변의 길이를 나타낼 때 쓰는 2i에서 i이다.

출력

첫째 줄에 필요한 큐브의 개수의 최솟값을 출력한다.

만약 채울 수 없다면 -1을 출력한다.

제한

  • 1 ≤ length, width, height ≤ 106
  • 1 ≤ n ≤ 20
  • 0 ≤ Ai < 20
  • 0 ≤ Bi ≤ 106
  • Ai ≠ Aj (i ≠ j)

예제 입력 1

4 4 8
3
0 10
1 10
2 1

예제 출력 1

9

예제 입력 2

4 4 8
3
0 10
1 10
2 10

예제 출력 2

2

예제 입력 3

10 10 11
1
0 2000

예제 출력 3

1100

예제 입력 4

10 10 11
1
0 1099

예제 출력 4

-1

예제 입력 5

37 42 59
6
0 143821
1 14382
2 1438
3 143
4 14
5 1

예제 출력 5

5061

출처

  • 문제를 번역한 사람: baekjoon
  • 문제의 오타를 찾은 사람: ho94949

알고리즘 분류

length × width × height 크기의 박스

이 박스를 큐브로 채우려고 한다.

큐브의 크기는 정육면체 모양이며 한 변의 길이는 2의 제곱 꼴 1, 2, 4, 8…

박스를 채우는데 필요한 큐브의 최소 개수를 찾는 문제

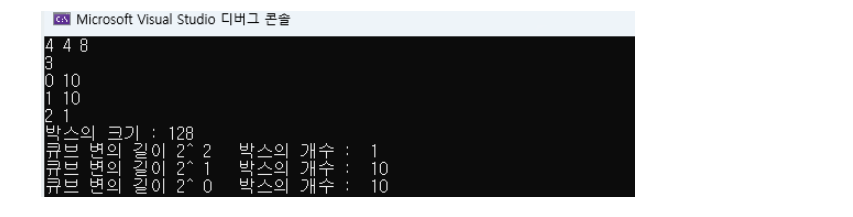
4 4 8 // 박스 length × width × height

3 // 큐브의 개수

0 10 // 변의 길이 : 2^0 10개 넓이 1 10개

1 10 // 변의 길이 : 2^1 10개 넓이 8 10개

2 1 // 변의 길이 : 2^2 1개 넓이 64 1개

넓이 128 64 + 10 + 10 + 10 + 10 + 10 + 10

박스는 부피

큐브는 각 큐브의 한 변의 길이와 개수만 가지고 있으면 될 것 같다

박스가 큐브의 개수와는 상관없이

만들어질 수 있는 경우의 수를 조건과 비교하면서 하면 어떨까???

입력 확인

#include <iostream>
#include <set>

using namespace std;

// 큐브의 변의 길이 순으로 내림차순 정렬
set<int, greater<int>> cubeSet;
set<int, greater<int>> ::iterator iter;

// 큐브의 개수를 저장 / 인덱스는 2^index
int cubeNumber[21] = { 0 };

// 박스의 가 세 높
int length, width, height = 0;

// 박스의 부피
int boxArea = 0;

// 큐브의 종류 , 큐브의 수
int type, Number = 0;

void Initializaion()
{
	cin >> length >> width >> height;
	boxArea = length * width * height;
	int N = 0;
	cin >> N;
	while (N > 0)
	{
		cin >> type >> Number;
		cubeSet.insert(type);
		cubeNumber[type] += Number;
		N--;
	}
}

int main()
{
	Initializaion();
	cout << "박스의 부피 : " << boxArea << "\n";
	for (iter = cubeSet.begin(); iter != cubeSet.end(); iter++)
	{
		cout << "큐브 변의 길이 2^ " << *iter << "   박스의 개수 :  " << cubeNumber[*iter] << " \n";
	}
    
	return 0;
}

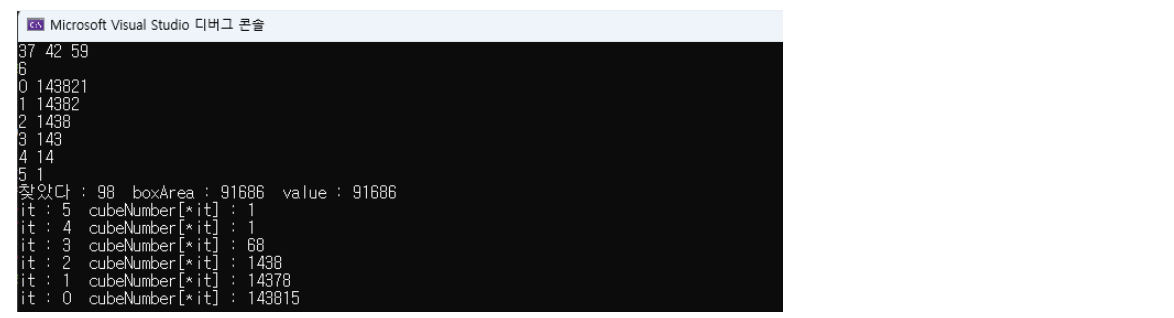
마지막 테스트 케이스에서 망… 함 ㅠ

부피로 접근하는 방법은 잘못된 방법이다

큐브들의 부피가 박스의 부피와 같다고 큐브가 박스 모양일 거라는 착각…

잘못된 로직과 코드 1

#include <iostream>
#include <set>
#include <cmath>

using namespace std;

// 큐브의 변의 길이 순으로 내림차순 정렬
set<int, greater<int>> cubeSet;
set<int, greater<int>> ::iterator iter;

// 큐브의 개수를 저장 / 인덱스는 2^index
int cubeNumber[21] = { 0 };

// 박스의 가 세 높
int length, width, height = 0;

// 박스의 넓이
int boxArea = 0;

// 큐브의 종류 , 큐브의 수
int type, Number = 0;


void Initializaion()
{
	cin >> length >> width >> height;
	boxArea = length * width * height;
	int N = 0;
	cin >> N;
	while (N > 0)
	{
		cin >> type >> Number;
		cubeSet.insert(type);
		cubeNumber[type] += Number;
		N--;
	}
}

void CubeCheck(int cnt, int value)
{

	if (value == boxArea)
	{
		cout << "찾았다 : " << cnt << "  boxArea : " << boxArea <<  "  value : " << value << "\n";
		for (auto it = cubeSet.begin(); it != cubeSet.end(); it++)
		{
			cout << "it : " << *it << "  cubeNumber[*it] : " << cubeNumber[*it] << "\n";
		}
		exit(0);
	}

	for (auto it = cubeSet.begin(); it != cubeSet.end(); it++)
	{
		if (cubeNumber[*it] == 0 || value > boxArea )
		{
			return;
		}
		//cout << "cubeNumber[" << *it << "]  : " << cubeNumber[*it] << "\n";
		cubeNumber[*it]--;
		//cout << "cnt : " << cnt << " 들어갈 때 : " << value << " ";
		CubeCheck(cnt + 1, value + pow(pow(2,*it),3));
		cubeNumber[*it]++;
	}
	cout << -1;
	exit(0);
}

int main()
{
	Initializaion();
	CubeCheck(0, 0);
	return 0;
}

그렇다면 박스에서 접근해야 한다.

박스를 큐브의 규격을 기준으로 분할하여 그리디 알고리즘을 적용하면서 푸는 것 인가?

잘못된 로직과 코드 2

#include <iostream>
#include <set>
#include <cmath>
#include <algorithm>

using namespace std;

// 큐브의 변의 길이 순으로 내림차순 정렬
set<int, greater<int>> cubeSet;
set<int, greater<int>> ::iterator iter;

// 큐브의 개수를 저장 / 인덱스는 2^index
int cubeNumber[21] = { 0 };
int cubeNeedNumber[21] = { 0 };

// 박스의 X,Y,Z
int BoxXYZ[3] = { 0 };
int temp[3] = { 0 };

// 큐브의 종류 , 큐브의 수
int type, Number = 0;

int cubeCNT = 0;

void Initializaion()
{

	cin >> BoxXYZ[0] >> BoxXYZ[1] >> BoxXYZ[2];
	sort(BoxXYZ, BoxXYZ + (sizeof(BoxXYZ)/sizeof(BoxXYZ[0])));
	int N = 0;
	cin >> N;
	while (N > 0)
	{
		cin >> type >> Number;
		cubeSet.insert(type);
		cubeNumber[type] += Number;
		N--;
	}
}

// 가장 큰 X를 기준으로 Z를 채워나감
// 들어오는 값은 X의 길이/Z의 남은 길이/ 몇번 나누었는지 확인(0부터 시작)
// 이 함수의 목적은 Z가 0이 될때까지 큐브를 찾는 것
void CubeCheckZ(int N, int leftZ, int divisionCount, int st)
{

	for (;leftZ >= pow(2, N);)
	{
		cout << "leftZ : " << leftZ << " pow(2, N) : " << pow(2, N) << "\n";
		if (divisionCount == 0 && st == pow(2, N))
		{
			BoxXYZ[2] -= st;
			st = -1;
		}
		
		if (st == -1)
		{
			cubeNeedNumber[N] += pow(4, divisionCount);
		}
		else
		{
			cubeNeedNumber[N] += pow(4, divisionCount) * (st / pow(2, N));
		}

		leftZ -= pow(2, N);
		
		if (leftZ == 0)
		{
			return;
		}
	}

	// 남은 Z의 길이보다 pow(2, N)가 더 큰 상황 
	for (;leftZ < pow(2, N);)
	{
		N--;
		divisionCount++;
		cout << "남은 Z의 길이보다 작은 박스 값 찾기 : " << " N : " << N << "  pow(2, N) : " << pow(2, N) << "  leftZ  :  " << leftZ << "\n";
	}

	CubeCheckZ(N, leftZ, divisionCount, st);
}


// 박스 전체의 X길이와 Z의 길이
void CubeCheckX(int BoxX, int BoxZ, int st, int boxY)
{
	if (BoxX == 0)
	{
		return;
	}
	// 처음 할 때
	int N = 0;
	for (int i = 0; i <= 20; i++)
	{
		if (boxY < pow(2, i))
		{
			N = i - 1;
			break;
		}
		else if (boxY == pow(2, i))
		{
			N = i;
			break;
		}
		
		//X의 값에 가장 근접한 2^i 를 구함
		if (BoxX < pow(2, i) )
		{

			N = i - 1;
			cout << "높을때 : " << N ;
			//cubeNeedNumber[N]++;
			break;

		}
		else if (BoxX == pow(2, i) )
		{
			//cout << "BoxX : " << BoxX << " 같을때" << N;
			N = i;
			//cubeNeedNumber[N]++;
			break;
		}
	}
	
	if (st <= pow(2,N))
	{
		st = pow(2, N);
	}

	// 박스의 Z길이보다 작거나 같은 2^N 의 N / 박스의 Z 길이 
	BoxX -= pow(2, N);
	CubeCheckZ(N , BoxZ, 0, st);
	cout << "BoxX  :  " << BoxX << "\n";
	CubeCheckX(BoxX, BoxZ, st, boxY);
}





int main()
{
	Initializaion();

	for (;BoxXYZ[2] > 0;)
	{
		CubeCheckX(BoxXYZ[0], BoxXYZ[1], 0, BoxXYZ[2]);
		cout << "BoxXYZ[2] : " << BoxXYZ[2] << "\n";
	}


	for (iter = cubeSet.begin(); iter != cubeSet.end(); iter++)
	{
		cout << "cubeNeedNumber[" << *iter << "]  : " << cubeNeedNumber[*iter] << "\n";

	}
	return 0;
}

잘못된 로직과 코드 3

작성하다 보니 내가 왜 이렇게 작성했는지 이해할 수 없다.

#include <iostream>
#include <set>
#include <cmath>
#include <algorithm>

using namespace std;

set<int, greater<int>> cubeSet;
set<int, greater<int>> ::iterator iter;

int cubeNumber[21] = { 0 };
int cubeNeedNumber[21] = { 0 };

long long BoxXYZ[3] = { 0 };

int type, Number = 0;

void Initializaion()
{

	cin >> BoxXYZ[0] >> BoxXYZ[1] >> BoxXYZ[2];
	
	sort(BoxXYZ, BoxXYZ + (sizeof(BoxXYZ)/sizeof(BoxXYZ[0])));
	int N = 0;
	cin >> N;
	while (N > 0)
	{
		cin >> type >> Number;
		
		cubeSet.insert(type);
		cubeNumber[type] += Number;
		N--;
	}
}

void CubeCheckZ(long long N, long long leftZ, long long divisionCount, long long MaxX, bool firstCheck)
{

	for (;leftZ >= pow(2, N);)
	{
		if (MaxX == pow(2, N) || leftZ >= pow(2, N) && firstCheck)
		{ 
			if (N == 0)
			{
				cubeNeedNumber[N] += leftZ;
				leftZ = 0;
				continue;
			}
			cubeNeedNumber[N] += pow(4, divisionCount);
			cout << "pow(4, divisionCount) : " << pow(4, divisionCount) << "  divisionCount  :  " << divisionCount << "  N :   "<< N << "\n";
			leftZ -= pow(2, N);
			continue;
		}

		if (leftZ >= pow(2, N) && !firstCheck)
		{
			if (N == 0)
			{
				cubeNeedNumber[N] += leftZ * MaxX / pow(2, N);
				leftZ = 0;
				continue;
			}
			cubeNeedNumber[N] += MaxX / pow(2, N);
			cout << "MaxX / pow(2, N) :  " << MaxX / pow(2, N) << "  " << MaxX << "  /  " << pow(2, N) << "\n";
			leftZ -= pow(2, N);

		}
	}

	if (leftZ == 0)
	{
		cout << "leftZ :  " << leftZ << "\n";
		return;
	}


	while(leftZ < pow(2, N))
	{
		N--;
		divisionCount++;
	}
	CubeCheckZ(N, leftZ, divisionCount, MaxX, firstCheck);
}

void CubeCheckX(long long BoxX, long long BoxZ, long long MaxX, long long boxY, bool firstCheck)
{
	if (BoxX == 0)
	{   
		BoxXYZ[2] -= MaxX;
		return;
	}

	int N = 0;
	for (int i = 0; i <= 20; i++)
	{
		if (BoxX < pow(2, i) || (BoxXYZ[2] < pow(2, i)))
		{
			N = i - 1;
			BoxX -= pow(2, N);
			break;
		}
		else if (BoxX == pow(2, i) || (BoxXYZ[2] == pow(2, i)))
		{
			N = i;
			BoxX -= pow(2, N);
			break;
		}
	}
	
	if (MaxX < pow(2,N))
	{
		MaxX = pow(2, N);
	}
	
	CubeCheckZ(N , BoxZ, 0, MaxX, firstCheck);
	firstCheck = false;
	CubeCheckX(BoxX , BoxZ, MaxX, boxY, firstCheck);
}

int main()
{
	Initializaion();
	
	while (BoxXYZ[2] != 0)
	{
		CubeCheckX(BoxXYZ[0], BoxXYZ[1], 0, BoxXYZ[2], true);
		cout << BoxXYZ[2] << " \n";
	}

	for (iter = cubeSet.begin(); iter != cubeSet.end(); iter++)
	{
		cout << "cubeNeedNumber[" << *iter << "]  : " << cubeNeedNumber[*iter] << "\n";
	}

	return 0;
}

통과된 코드

위와 같이 분할 된 박스를 다시 분할 X, Y, Z 가 0일 때까지

상자의 개수 조건에 부합하지 않으면 체크하고 -1 출력

#include <iostream>
#include <map>
#include <cmath>

using namespace std;

//cubeMultimap 은 내림차순으로 정렬된다. 
multimap<int, int, greater<int>> cubeMultimap;
//cubeMultimap 순회를 위한 반복자
multimap<int, int, greater<int>>::iterator iter;


int length, width, height;
int numberOfCubes, cnt = 0;
int tempOne, tempTwo;
bool failCheck = false;

// 조건을 초기화 해주는 함수
void Initializaion()
{
	cin >> length >> width >> height >> numberOfCubes;
	while (numberOfCubes > 0)
	{
		cin >> tempOne >> tempTwo;
		cubeMultimap.insert(make_pair(pow(2, tempOne), tempTwo));
		numberOfCubes--;
	}
}

// 분할 정복을 위한 함수 (X, Y, Z, 반복자)
void divisionConquest(int length, int width, int height, multimap<int, int, greater<int>>::iterator iter)
{
	// 만약 X, Y, Z 중에서 0이 되는 값이 있다면 리턴
	if (length == 0 || width == 0 || height == 0) return;

    // 분할정복 로직부분
	for (;iter != cubeMultimap.end(); iter++)
	{
		if (iter->second != 0 && length >= iter->first && width >= iter->first && height >= iter->first)
		{
			iter->second--;
			cnt++;
			divisionConquest(iter->first, iter->first, width - (iter->first), iter);
			divisionConquest(length - iter->first, height, width, iter);
			divisionConquest(height - iter->first, width, iter->first, iter);
			return;
		}
	}

	// 만약 큐브의 조건이 만족하지 않는다면 실패 마킹
	failCheck = true;
	return;
}


int main()
{

	Initializaion();

	// cubeMultimap의 가장 높은 값부터 시작
	iter = cubeMultimap.begin();
	divisionConquest(length, width, height, iter);

	// 실패 마킹이 true 면 항상 -1 출력
	if (failCheck) cout << -1;
	else cout << cnt; // 아니면 큐브의 개수를 출력한다.

	return 0;
}

분할 정복의 개념과 구현 방법은 몰라서 오래 고민한 문제

재귀 구현 어려웠다.

댓글 달기

이메일 주소는 공개되지 않습니다. 필수 필드는 *로 표시됩니다

관련 게시물

위로 스크롤